How to Convert Units
Learn how to use the multiplicative identity to help you convert units from miles to kilometers, kilometers to light-years, and more.
In the last article, we began discussing how to convert from one system of units to another—for example from miles to kilometers—and how all of this relates to the multiplicative identity. But we didn’t quite have enough time to get to the punch line, so without further ado let’s finish up and get to the bottom of exactly how to convert between units.
We started the last article off by talking a bit about the power of measurement—in particular how quantitative measurements (that is, measurements with numbers) give us information about the world. We also talked about the units we use when making measurements. For example, when you measure the length of something, perhaps the diagonal size of your precious new television (just to make sure you got what you paid for), you can’t just say the length is 50. That number 50 alone doesn’t tell us anything about the size of your TV since we don’t know 50 of what. Are we talking miles? Meters? Thumb lengths? You have to give the units you used to make the measurement for it to make any sense. In this case, you measured 50 inches—which now makes perfect sense!
We also spent some time in the last article talking about the multiplicative identity. We found out that the multiplicative identity is really just a fancy word for the number 1. The multiplicative identity property says that you can multiply any number by the multiplicative identity, 1, and the answer will be the same number. No big surprise there, I know—so how is this all related to converting units? Well, let’s find out.
Let’s imagine that after measuring the size of your new TV screen, 50 inches, you can’t help but wonder if that’s bigger than your little brother. So you ask your brother how tall he is, and he tells you that he’s 4 feet tall. That doesn’t immediately give you the answer though—you still need to figure out how to convert 4 feet into inches so that it can be directly compared to the 50 inch size of your TV. (I know that many—or most—of you will already know how to do it; that’s fine—it’s the method that’s important here, not the specific numbers or choice of units we’re using.)
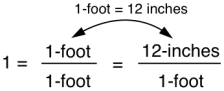
So here’s what we’re going to do: Let’s write a fraction that’s equal to the number 1. What does that look like? Well, any fraction where the numerator and denominator are the same number must equal 1. For example, 2/2 and 4/4 are both equal to 1. And that makes perfect sense since putting together 2 halves or 4 quarters of something must give a whole. Okay, how else can we make a fraction that’s equal to 1? How about something like the fraction: 1-foot / 1-foot? The numerator and denominator are the same, so even though there are units in it this fraction must equal 1 too! Okay, here comes the important part. How many inches are in 1 foot? 12 inches-per-foot, right? Indeed, which means that the fraction 12-inches / 1-foot is equal to 1!
Now this is getting interesting. Since 1 is equal to the fraction 12-inches / 1-foot, and the multiplicative identity property says that we can multiply any number by 1 without changing its size, that means we can multiply the height of your little brother, 4 feet, by the fraction 12-inches / 1-foot to get his height in inches. In other words, let’s write the problem as 4-feet times the fraction 12-inches / 1-foot.
That is, we have 4-feet and 12-inches multiplied together in the numerator, and the length 1-foot in the denominator. Now, the units of feet on the top cancel with the units of feet on the bottom, leaving us with the problem 4 x 12 inches, which is just 48 inches.Remember, all we did to get the answer was multiply by the number 1—we just used a peculiar but very handy version of that number 1. And, as it turns out, your brother is 48 inches tall—that’s 2 inches shorter than your new TV!
How to Convert Distance Units
The quick and dirty tip for converting from unit-1 to unit-2 is to multiply the number of unit-1s by the fraction that gives the number of unit-2s over, or per unit-1 (check out the article “How to Multiply Fractions” for a refresher on how to do this). In other words, in the problem of converting the height of your little brother from feet to inches, we multiplied the number of feet (the unit-1s, which in the example was 4) by the fraction giving the number of inches-per-foot (the number of unit-2s per unit-1, which in the example was 12/1).
[[AdMiddle]So, to convert from miles to kilometers—say you want to know how many kilometers there are in 7 miles—you just need to know the number of kilometers-per-mile. You’ll then create the fraction #-of-kilometers / 1-mile (“the number of kilometers per 1 mile”), and multiply it by the number of miles you’re measuring—in this case, 7. Or, to convert from light-years to kilometers, you just need to know the fraction giving the #-of-kilometers / 1-light-year, and then multiply the number of light-years by this fraction to find the number of kilometers. It’s not too difficult…except for one thing: How do you find out what these fractions are—like the #-of-kilometers per 1-light-year?
Well, you’re in luck because Google can help with that. Life didn’t used to be so easy, but now all you have to do is google something like “kilometers per light year” to find that 1 light-year is about 9.46 x 1012 kilometers—which is a lot of kilometers—almost 10-million million of them! (We’ll talk more about how to read and use this type of “scientific notation” in a future article.) So what’s the fraction for #-of-kilometers / 1-light-year? According to Google, it’s 9.46 x 1012 kilometers / 1-light-year. That’s all there is to it!
mailto:mathdude@quickanddirtytips.comcreate new emailhttps://www.youtube.com/user/jasonmarshallTVhttps://twitter.com/jasonmarshallhttps://www.facebook.com/TheMathDude
About the Author
Jason Marshall is the author of The Math Dude’s Quick and Dirty Guide to Algebra. He provides clear explanations of math terms and principles, and his simple tricks for solving basic algebra problems will have even the most math-phobic person looking forward to working out whatever math problem comes their way.
