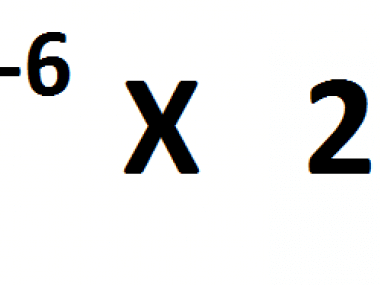What Is Exponential Growth?
Have you ever heard that something is growing exponentially? Or that it’s accelerating at an exponential pace? What does exponentially really mean?
This article was updated and republished on March 25, 2020. Transcript text may not match the podcast audio.
A while back, I ran across a tweet from B.J. Novak (aka, Ryan from TV’s The Office) stating: “The misuse of the word ‘exponentially’ has increased dramatically.”
If you know what the word “exponentially” actually means, then you probably agree with Mr. Novak’s observation that there has been a dramatic (although not truly exponential) increase in the misuse of this word. Nowadays, people utter “exponentially” as another way of saying “rapidly” (or perhaps “really rapidly”). But mathematically, that simplifies the definition a little too much.
So, what does exponential actually mean, math-wise? What are some actual, non-hyperbolized examples of real word exponential growth? And how can you save yourself from making this mathematical faux pas? (Or is it even a faux pas at all?)
An exponential increase in “exponentially”?
If something is growing exponentially, it’s growing rapidly … very rapidly. But it’s not just that—it’s growing rapidly in a precise and predictable way. At least according to the proper mathematical definition of the word (more on exactly what that is in a minute).
If something is growing exponentially … it’s growing rapidly in a precise and predictable way.
Of course, these days people are a lot more relaxed with the word. Take, for example, Twitter user and NBC New York reporter @Checkey4NY’s recent tweet stating that “Crowds on 5th Ave have literally grown exponentially in just a few minutes.” I particularly like this tweet since it includes both the newfangled version of “exponentially” as well as the often funny word “literally” (because it’s so frequently used to mean figuratively). In other news, sportscaster @SportsBoyTony noted in his recent tweet that “[Collinsworth] gives you exponentially more than Simms.” And finally, in a totally different vein, Twitter user @iMuggle recently tweeted “I just remembered we are having sushi for dinner. [The] day just got exponentially better.”
If you know the actual mathematical meaning of the word “exponentially,” you’ll recognize that these folks don’t really mean exponentially. If they did, well, that’d just be crazy. Why crazy? Let’s find out the precise mathematical meaning of the word.
The mathematical meaning of “exponentially”
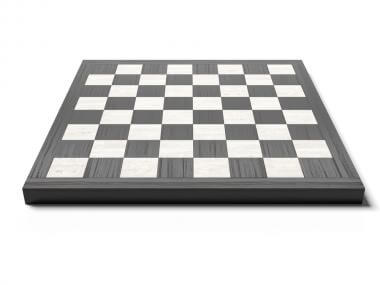
To understand what math says about actual exponential growth, let’s recount the 1,000-year-old story of the rice and chessboard problem that we first discussed when figuring out the odds of predicting a perfect NCAA tournament bracket. According to this story, the emperor of India was so impressed with the inventor of some new game called chess that he granted him any reward he wished for. Being clever, the inventor asked only to receive 1 grain of rice for the first square of his chessboard, 2 for the second, 4 for the third, 8 for the fourth, and so on doubling the number of grains for each of the 64 squares of his board.
Thinking this a meager request, the emperor agreed and was left rather pleased with himself—right up until somebody ran the numbers and pointed out that the final square of the chessboard would contain 263 grains of rice, which is a lot of rice! In fact, the 264 – 1 total grains of rice on the chessboard would end up forming a mountain of rice larger than Mt. Everest. Indeed that’s a lot, lot, lot of rice.
And that’s what real exponential growth looks like. In this case, the number of grains of rice on each square grows as 2n, where n is the number of the square (starting with n=0 for the first square and going up to n=63 for the last). This means that each successive square has twice as many grains as the previous one! This type of growth isn’t just kind of speedy, it’s a kind of speedy that gets increasingly speedy as you go along. A bit more precisely, if something is increasing exponentially like this, its rate of growth (in other words how fast it’s growing) must be proportional to its current value. This means the bigger it gets, the more rapidly it gets even bigger … and on and on.
Real world exponential growth
Exponential growth is a pretty good description of how colonies of humans grow. The more humans there are, the more humans there are to reproduce and make more humans—so the rate of growth is related to the size of the population. It also describes the way a virus spreads. The more people who become infected with a virus, the more people there are to spread it and make others infected.
Other examples include the way that an uncontrolled nuclear chain reaction grows, the way that compound interest grows, the way that Moore’s law tells us computer processing power grows over time, and lots of other stuff, too.
Exponential growth is a pretty good description of how colonies of humans grow. It also applies to the spread of viruses.
So true mathematical exponential growth is fairly common in the real world, just not in the way that people think.
Is this an exponential misuse?
I’d like to finish up with one final thought: Is the misuse of the word “exponentially” really a misuse? This is probably a question best left to Grammar Girl, but it seems to me that if people want to use, misuse, or metaphorically repurpose the word “exponentially,” then it’s their right to do so. And I guess that means I have to resign myself to the fact that in addition to its precise mathematical meaning, “exponentially” now also just means “super-duper fast.”
I suppose this really isn’t a big deal, and in truth, it’s something I’ve had to make peace with before since this sort of double-meaning exists all over the place—particularly wherever technical meanings cross paths with colloquial ones. Case in point, people use the word “theory” all the time to talk about their conjectures, guesses, or even hog-wild speculations about how something works or why it’s happening. But in science, the word theory means something altogether different: theories in science are the most scrutinized, tested, and well-established ideas that we have. They’re sort of the complete opposite of the thing people commonly toss around as a theory (which explains why so many people get tripped up thinking that scientific theories are “just theories”).
While these multiple-uses of words are no-doubt a wee-bit unfortunate, they’re also here to stay. So suffice it to say that my theory is that these misuses and reuses will continue growing exponentially, and thus are simply something we have to deal with.
You May Also Like…
About the Author
Jason Marshall is the author of The Math Dude’s Quick and Dirty Guide to Algebra. He provides clear explanations of math terms and principles, and his simple tricks for solving basic algebra problems will have even the most math-phobic person looking forward to working out whatever math problem comes their way.
